Sectieoverzicht
-
A fractional exponent is the same as finding some root of a number. We generalise this as: \({x }^{\frac{1}{n}}= \sqrt[x]{n}; n\in N\ , x\in R\).
The root symbol has a special name, it is called a radical. Each part of a radical has its own name, as shown below.
Rewriting fractional exponents as radicals
To change a fractional exponent to a radical, rewrite the number in the numerator of the exponent as the exponent of the radicand, and the number in the denominator of the exponent as the root as shown in the image below.
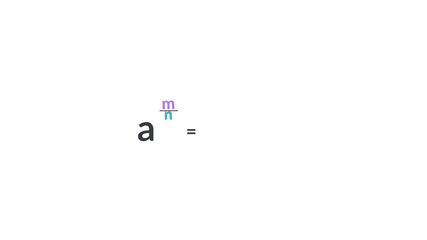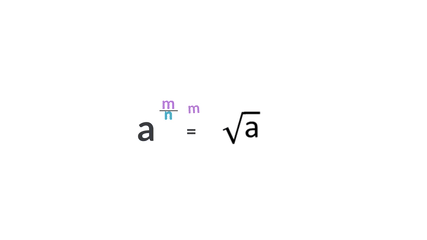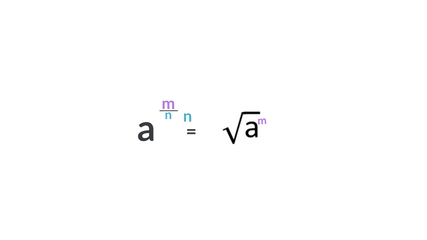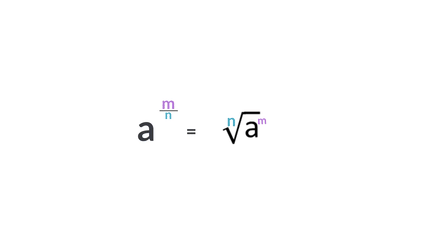
Test your skills of changing from fractional exponents to radicals by trying the next activity.
-
Leerlingen moetenAls voltooid aanduiden
Activity: Rewriting rational exponents
-
Leerlingen moetenAls voltooid aanduiden
Rationalising denominators
Surds are roots that cannot be reduced to a whole number or fraction. When a surd is in the denominator of a fraction we need to remove the surd to make the fraction simpler to work with.
The process we use to remove a surd from the denominator is called rationalising the denominator. This concept is shown in the next video.
-
-